What Is a Spherical Cap? (With Visual Analogy)
At first glance, the term spherical cap might sound a bit technical—but chances are, you’ve seen one today without even realizing it. A spherical cap is simply the portion of a sphere that’s been sliced off by a flat plane. Imagine taking a perfectly round ball and slicing the top off with a knife. That curved piece you’ve removed? That’s your spherical cap.
To make it even more relatable:
Think of the top of a dome, the surface of a contact lens, or the curved crown of a helmet. All of these are real-life examples of spherical caps. Even the rounded edge of a bowl or the top half of a scooped melon fits the description.
Try out Ellipsoid Volume Calculator
How to Calculate the Volume of a Spherical Cap?
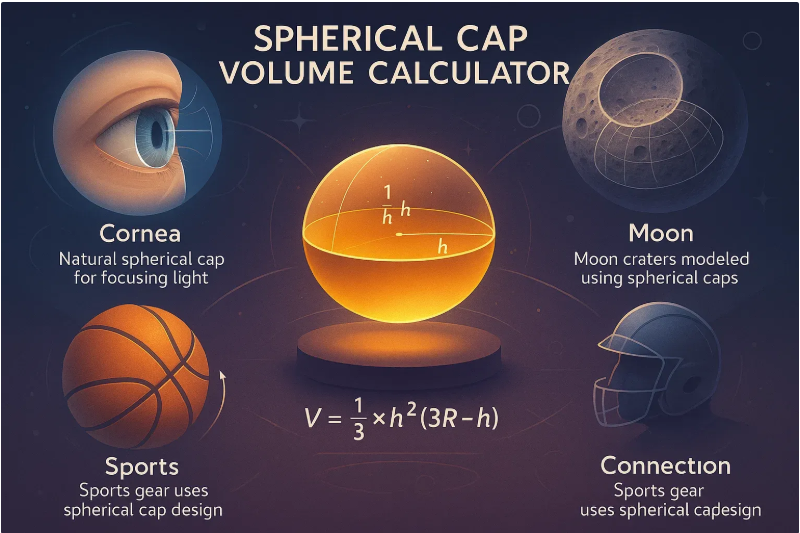
Now that you know what a spherical cap is, let’s get into the math. Don’t worry—it’s just one formula, and once you understand what each part means, it’s surprisingly straightforward.
The volume of a spherical cap is given by this formula:V=13h2(3R−h)
Where:
-
Vis the volume of the spherical cap -
his the height of the cap (from the flat base to the curved top) -
Ris the radius of the original sphere
This formula comes from integrating a segment of the sphere in three dimensions—but you don’t need to dive into calculus to use it. You just plug in your values for h and R, and let the math do its thing.
Of course, if you're not in the mood to calculate it by hand every time (and we don’t blame you), feel free to use our free Sphere Volume Calculator. It handles the formula instantly and helps you double-check your math—great for students, professionals, or the just-plain-curious.
From Ancient Astronomy
The concept of spherical caps isn’t modern—it dates back to ancient times. Greek astronomers like Hipparchus used spherical geometry to model the heavens, imagining planets embedded in rotating spheres. Later, during the Islamic Golden Age, scholars like Alhazen applied similar math to optics, using spherical sections to understand light and vision.
Even in architecture, this geometry made an impact. Antoni Gaudí, for example, used spherical cap shapes to design the domes of Sagrada Família, blending beauty with structural strength.
In short, whether in stargazing, science, or design, spherical caps have long helped us make sense of the curved world around us.
Try out Cylinder Volume Calculator
Fun Facts About Spherical Caps
💡 Fun Fact: Your eye’s cornea is a natural spherical cap. That curved surface is precisely what helps focus light, which is why vision correction, like contact lenses or LASIK—requires extremely accurate curvature measurements.
💡 Fun Fact: Many of the Moon’s craters are modeled as spherical caps in space studies. Scientists use this geometry to estimate crater volume, study impact events, and even predict what’s beneath the surface.
💡 Fun Fact: In sports, spherical caps show up more than you think. The rounded tip of a basketball, football, or even some helmets follows the same geometry—offering both aerodynamic form and visual symmetry.
Spherical caps might seem like obscure math, but they’re all around us—quietly shaping how we see, play, and explore the universe.
Check out Math section to solve math quick and easy

